Algebra Tiles – Maths Materials

Algebraical Experience Material or Algebra Blocks

• NUMBER: Integers

• Algebra

W.W. Sawyer developed the idea behind Algebra Tiles in the 1940s. Z.P. Dienes worked with them in the 1960s and Charles Lovitt and colleagues published a manual about them in the 1970s.

Algebra Tiles are a concrete, visual, area-based model which naturally link students’ previous understandings to algebraic representations, forging the links of factors and the distributive property from number and applying them to algebra.

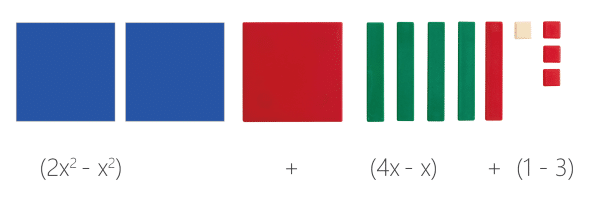
Mathematical Language

Expand, factorise, integer, simplify, square, negative numbers.

Using Algebra Tiles

Using the simple properties that a positive added to a negative is equal to zero, and that you can add or subtract zero without changing the value of an expression, algebra tiles build on students’ conceptual understandings by applying number laws to algebraic expressions.

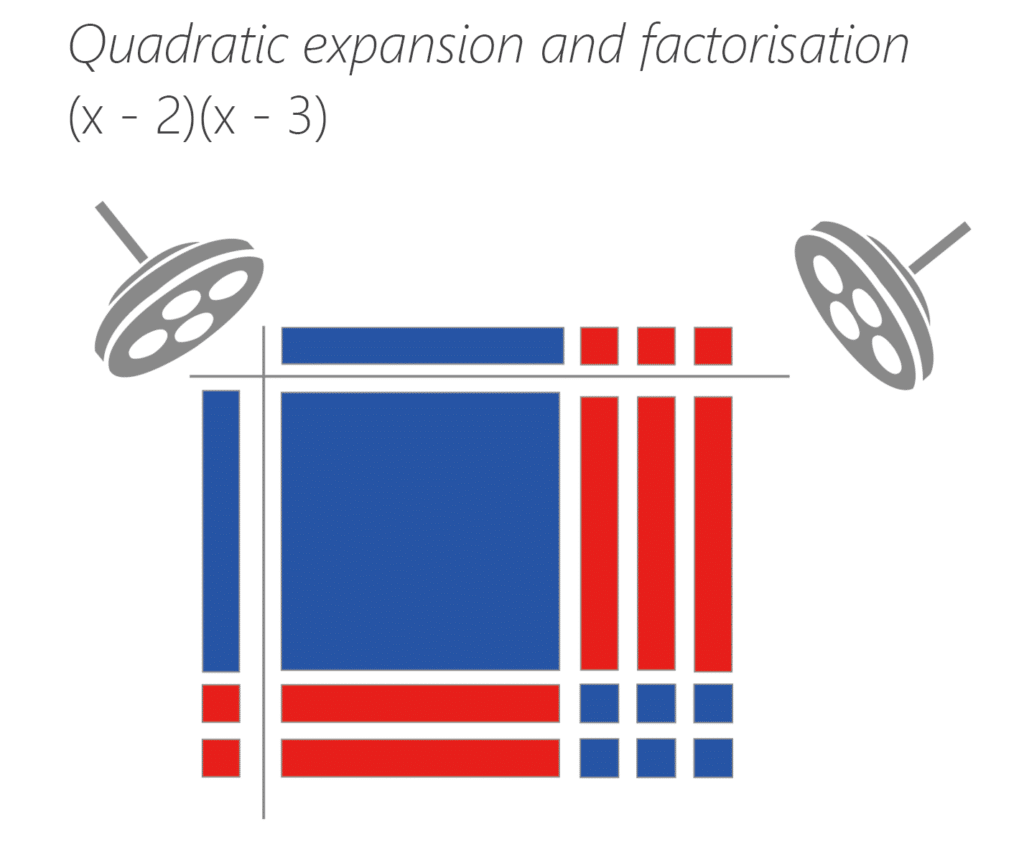
Typical Classroom Requirements

Generally Algebra Tiles are used at the top end of primary/elementary school and in lower secondary school to help students understand some fundamental algebra ideas.

A class set:

– 16 wallets of Algebra Tiles.